Sonnack.com

Short, but very, very fast!

The last two train examples (Lightning Strikes and Treaty Train) focused on how simultaneity is relative to motion. Our final train example focuses on how length is relative to motion. The faster something goes relative to you, the more it appears foreshortened along its direction of travel.

This example involves a train that, if it stopped halfway through, is too long for a tunnel — it would stick out both ends. But motion contracts length, so if the train goes fast enough, it becomes short enough to fit entirely inside the tunnel.

And it's not an illusion; the train really does fit inside!

When the train seems entirely inside the tunnel, it's possible to imagine briefly closing large doors at either end of the tunnel.^[1] If the doors enclose the train without chopping part of it off, then the train somehow "really is" shorter due to its (very high speed) motion.

The interesting thing is that — from the train's point of view — the tunnel is speeding past, so the tunnel is foreshortened. That makes the train seem even longer compared to the tunnel.

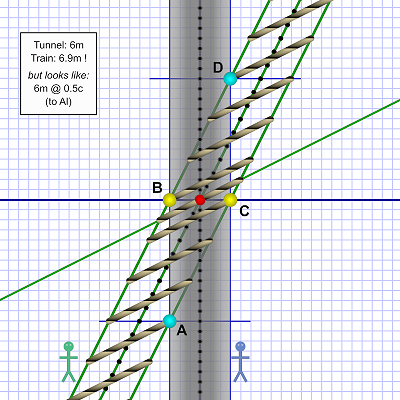
Here's what the scenario looks like from Al's on-the-ground frame of reference:

Diagram 1. Al's view of Em (and train) going through tunnel.

Al watches as the train (carrying Em) approaches from the left, goes through the tunnel, and keeps on going to the right. They agree to call the moment Em is halfway through the tunnel the mutual origin (red dot).

Event A marks the moment the front of the train enters the tunnel, and event D marks the moment the rear of the train exits the tunnel. Between those events, some part of the train is in the tunnel.

Since the train is moving (very fast) from Al's perspective, it appears foreshortened to him. In this case, the train is now short enough to fit entirely inside the tunnel.

Really fast doors!

At the moment it does, Al presses a button that briefly closes doors over both ends of the tunnel (events B & C). The doors re-open in time for the train to continue on its way without stopping or slowing down.

Logically, the doors close when Em is halfway through the tunnel — the point they've agreed to call the origin of their individual coordinate systems. So the doors close simultaneously with the origin… from Al's perspective.

Think about it: For a moment, from Al's point of view, a train he knows is too long for the tunnel fits entirely within the tunnel due to length contraction along its line of travel. Somehow, it really does fit — that Al can close both doors proves it!

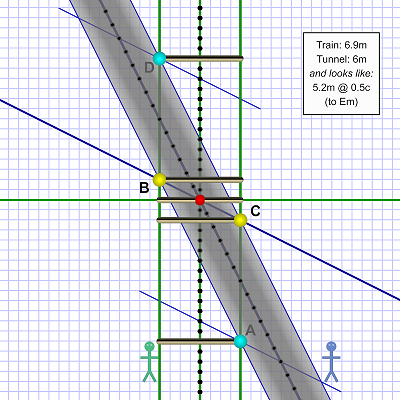
Now, here's what things look like from Em's in-the-train frame of reference:

Diagram 2. Em's view (from train) of Al and tunnel passing them.

Em (on the train) watches as the tunnel speeds towards her from the right. It rushes past her (and the train) and keeps on going to the left. Three distinct moments occur for Em:

¶ The tunnel's exit reaches the front of the train (event C). Just before it does, the exit door shuts and re-opens. Because the train is longer, the rear end still sticks out of the tunnel. In fact, the midpoint of the tunnel hasn't reached Em yet (her clock reads t=-2).

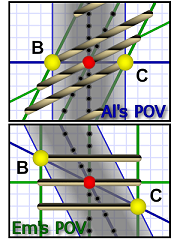Two views of Em passing the origin (tunnel midpoint).

¶ Two ticks later the tunnel midpoint reaches Em (making this moment t=0). The tunnel exit has passed the front of the train while the tunnel entrance still hasn't reached the rear of the train! So at Em's t=0 — when she's halfway through the tunnel — from her point of view, the train is sticking out both ends!

¶ Two more ticks and the tunnel entrance reaches the train's rear end. As it passes, the door shuts and re-opens (event B).

This example is really a re-imaging of the Simultaneous Lightning Strikes example from Monday. In this case, instead of lightning strikes, doors close and open. These events are simultaneous to Al, but not to Em.

The key difference is that this example focuses on the length contraction of the train. It's relative only to Al's frame of reference, but the foreshortening allows him to briefly enclose the train entirely inside the tunnel.

Of course, Em has no awareness of being foreshortened! She feels perfectly normal.

But when she looks out the train window she sees Al, the tunnel, and the whole world zipping past, and they look foreshortened!

The mind twisting thing is this: If Em doesn't feel — and from her point of view she isn't — foreshortened, then how does the train fit in the tunnel (one that, from Em's point of view, is even shorter)?

It has to do with those lines of simultaneity. (You may be as tired of reading that phrase as I am of typing it, but it's fundamental to Special Relativity. You can't really understand SR without understanding surfaces (lines, planes, volumes) of simultaneity.)

Look at it this way: If the train did fit the tunnel and stopped inside, it would be simultaneous with the tunnel. Both would experience each instant together in parallel. (They'd make vertical lines on a T-S diagram.)

Imagine tiny clocks wallpapered to every surface. Everywhere you look, tiny clocks all keeping time together. (On a smaller scale, think how you can place a regular clock anywhere in a room and it keeps the same time.)

This idea of all the tiny clocks ticking away in synch together is what I mean about all points in your frame of reference going through time together. In our own frame we slice time into "horizontal snapshots" (exactly in reference to lines of simultaneity on time-space diagrams).

When we look at the points of a moving reference frame, they don't go through time in lockstep but in a progression. A moving frame "slices time" at an angle relative to ours.

From Al's point of view, at the t=0 origin moment, a complete train is inside the tunnel. As we saw, at Em's t=0 moment — where she's at the tunnel's midpoint, the coordinate (0x, 0t) origin — the train was sticking out both ends of the tunnel. We know those moments align because both put Em at the tunnel's midway point.

But Em's t=0 line of simultaneity is rotated out of Al's! Only the center, with Em at the origin point, is really in Al's zero moment (that is, in fact, what defines the mutual origin event for them — Em being at the origin point).

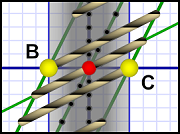Three snapshots of Em.

Consider the t=0 snapshot of the train: The front is in Al's later moments (above Al's t=0 line). It's already seen the exit door close and re-open (event C). From Em's perspective, the front of the train is out of the tunnel on its way. (Em reaches the origin point after event C according to her.)

Meanwhile, the back of the train hasn't even reached Al's t=0 moment yet. Em's says event B happens after she reaches the origin, so the entrance door closing hasn't happened yet.

So she has no problem with the tunnel being too short. The front of her train at that moment projects into Al's future (where the exit door is open again), while the rear drags behind in his past (where the entrance door hasn't closed yet).

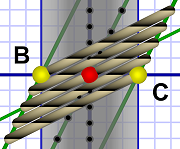Al traps slices of the train at various moments in time.

So what train does Al trap in the tunnel? Look at the diagram to the right. The exit of the tunnel and the front of the t=-2 train share event C. Likewise the entrance of the tunnel and the rear of the t=+2 train share event B. The midpoint of the t=0 train shares the origin point. This diagram adds train snapshots at +1t and -1t to fill things in.

So the left side of the tunnel contains a "later" train while the right side contains an "earlier" train. The further from center the more into Al's past or future the train extends.

That's enough to think about for now!

I'll wrap up with length contraction next time.