SR #12: Relative Simultaneity
 Flatland Al!
Flatland Al!We started by exploring the idea that motion is relative. Now we see that the idea of simultaneity is relative! Events that Al sees as simultaneous in his frame of reference do not appear simultaneous to Em — she sees them happening one after another!
A frame of reference has lines of simultaneity that allow us to assign time coordinates to events in the reference frame. If Al and Em have different lines of simultaneity, then their coordinate systems differ— they assign different coordinates to an event!
Let's explore that in a bit more detail…
We'll compare the situation from Al's frame of reference as well as from Em's. In each case we'll consider a set of events that appears simultaneous in one frame of reference but not another. Notice that each case is a mirror image of the other. Both Al and Em see themselves as motionless and the other as moving.
In the diagrams that follow, lines in Al's frame of reference are blue; lines in Em's are green. (Click on any of the diagrams below for a large version.)
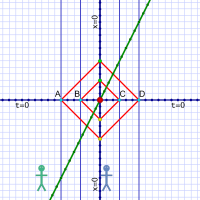 Diagram 1. Al's view of using his laser to measure events A–Z. Diagram 1. Al's view of using his laser to measure events A–Z. |
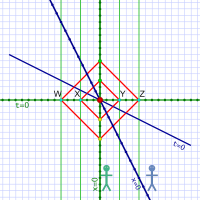 Diagram 2. Em's view of using her laser to measure events W–Z. Diagram 2. Em's view of using her laser to measure events W–Z. |
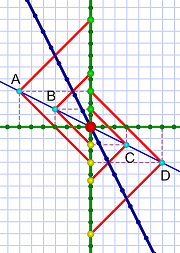
¶ Al (diagram 1) thinks he's standing still. He sees events A, B, C, & D, as simultaneous and defines that moment as his t=0. (Of course, his own world line — vertical blue line in center — is x=0.)
He sees Em moving past him (at c/2) from left to right (thick green line). They pass at their mutual origin point (0x, 0t).
Notice that Em's timer ticks (dots on her world line) are more widely spaced than Al's. From Al's perspective, Em's clock appears to be ticking slower.
¶ Em (diagram 2) thinks she's standing still. She sees different events W, X, Y, & Z, as simultaneous and defines her t=0 as the moment when they occur. (And her own world line — vertical green line in center — is x=0.)
She sees Al moving past her (at c/2) from right to left (thick blue line). They meet at (0x, 0t).
According to Em, Al's clock seems to be ticking slower, since his timer dots are further apart.
 Diagram 3. Al's view of Em using her laser to measure events A–D. Diagram 3. Al's view of Em using her laser to measure events A–D. |
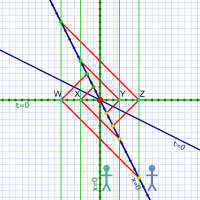 Diagram 4. Em's view of Al using his laser to measure events W–Z. Diagram 4. Em's view of Al using his laser to measure events W–Z. |
Here's what the motionless observer sees as the moving observer uses their lasers to map events that are simultaneous to the first observer. It's apparent that these light path patterns don't have "synchronicity symmetry." The moving observer doesn't see the events as simultaneous!
¶ In diagram 3, Al (blue line) watches Em measure his simultaneous events, A, B, C, & D, while she zips past (green line). He sees her laser light following a 45-degree path.
¶ In diagram 4, Em (green line) watches Al zip past (blue line) and measure her simultaneous events, W, X, Y, & Z. She sees his laser light following a 45-degree path.
 Diagram 5. Em's view of using her laser to measure events A–D. Diagram 5. Em's view of using her laser to measure events A–D. |
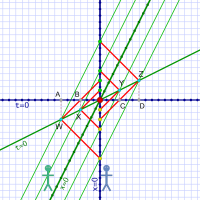 Diagram 6. Al's view of using his laser to measure events W–Z. Diagram 6. Al's view of using his laser to measure events W–Z. |
Now we're changing our perspective to what the observer who is moving (relative to the simultaneous events) sees in this situation. (The first pair of diagrams were from the perspective of an observer standing still relative to the events.)
¶ Diagram 5 is Em's view of Al and events A, B, C, & D, in diagram 3. Of course she also sees her laser light move at a 45-degree angle.
¶ Diagram 6 is Al's view of Em and events W, X, Y, & Z, in diagram 4. He, likewise, sees his laser light move at a 45-degree angle.
 No synchro-symmetry!
No synchro-symmetry!The lack of synchronicity symmetry is obvious. The laser start and end times don't match, and — more importantly — neither do the inflection (bounce) points. The moving observer does not see the events as simultaneous.
(The rotational symmetry around the origin point is due to the way the experiment is set up. The events are equally spaced, and Al and Em agree to call the point and moment they pass the origin of their coordinate system (0x, 0t). This makes the events shift in a regular way.)
Notice that, the further away the event is from x=0, to greater the difference in its apparent time.
High speed is a key aspect of Special Relativity, but when it comes to synchronicity, distance is also a factor. I've read that walking speed is enough to make events in the Andromeda Galaxy appear to have different time coordinates than when standing still!
(This is hard to calculate! The gamma factor for 5 MPH is a one followed by a decimal point and 20 zeros. Then there's a four (and two more zeros). On the other hand, the distance (in meters) to Andromeda is a number with over 20 digits, so it wouldn't surprise me if the math works out.)
Finally, to cover all the bases and bring it home, here's what the moving observer sees when the motionless observers do their original tests of simultaneity (diagram 1 & diagram 2):
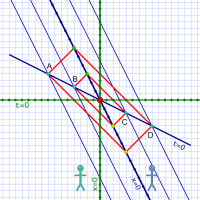 Diagram 7. Em's view of Al using his laser to measure events A–D. Diagram 7. Em's view of Al using his laser to measure events A–D. |
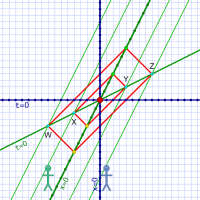 Diagram 8. Al's view of Em using her laser to measure events W–Z. Diagram 8. Al's view of Em using her laser to measure events W–Z. |
¶ Diagram 7 is Em's view of Al's symmetry test in diagram 1. She can see why Al thinks events A, B, C, & D, are simultaneous — to him, they are!
¶ Diagram 8 is Al's view of Em's symmetry test in diagram 2. He, likewise, can see why she sees events W, X, Y, & Z, as simultaneous.
The crucial point here is how the lines of simultaneity shift due to relative motion. And since our coordinate systems are based, in part, on the lines of simultaneity, that means our coordinate systems shift due to relative motion.
This means that how we map space and time changes when we move. The effect is incredibly tiny at the speeds we normally move (19 decimal places to see a difference), but it's there. At high speeds — Special Relativity speeds, it's much more obvious.
Next time I'll wrap up this section on simultaneity, and then we'll be ready to break down the infamous train thought experiments.
 Constant laser flashes!
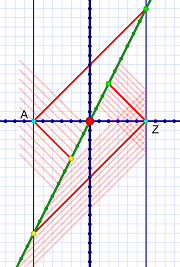
Constant laser flashes!As a last point: If you've wondered how Al or Em know when to fire their lasers in order to hit the events, good for you for thinking things through!
It turns out Al and Em constantly send laser flashes (in both left and right directions) and watch constantly for reflections.
If they encode into each flash the time they sent that particular laser flash, they can use that time stamp to identify the send time.
They record time when they see a reflection of a flash (the time stamp of which tells them when that flash was sent). They also record which direction (left or right) the flash came from.
There are two basic possibilities:
Event A doesn't reflect light except at the moment of the event. (A gopher pops his head up for a moment.) On the left side of the diagram above, laser flashes sent to A's position just pass it and keep on going. The only one reflected back is the one sent at the right moment. We identify the event by which flash was reflected.
Event Z reflects back each flash, but we only care about the flash that illuminates the event's moment (which just happens to also be event A's moment as well as t=0 in the diagram). We see and ignore the other flashes because they don't reflect the right moment in time.
The bottom line: While there are many flashes, the diagrams only show the ones where the light did hit the event at the right time.