Sonnack.com

Not once, but twice!

For the last three weeks I've been laying a firm groundwork for the more interesting part of the series. Perhaps there was too much time and detail: I seem to have lost much of my audience (not that the lecture hall was packed in the first place).

I've long believed in the importance of basic knowledge — it's stood me in good stead through life. But I know not everyone shares my appetite for details. For what it's worth, the rest is the fun part, where all that groundwork goes into action.

This week, trains; next week, spaceships!

I haven't yet gone into this in detail, but when you move at relativistic speeds (speeds fast enough for the effects of Special Relativity to become noticeable), others see three things happen to you:

1. Your clock runs slower (time dilation).
2. Your length along the direction of travel contracts.
3. Your mass increases.

I want to stress that this is what others — observers in different frames of reference — see happening to you. From  your point of view, everything seems normal. And since you see the others as moving, those things seem to be happening to them, not to you.

All observers think their own clocks run normally and have no perception of their own length contraction or mass increase. Observers only see those things happening to others who are moving relative to them.

Remember the first rule: The physics within a frame of reference is the same (i.e. normal) for all observers in that frame. Clocks and other measuring devices depend on physics to work. When you hold a foot-long hot dog, it's always twelve inches long no matter how fast you're going.

To someone watching you pass by, to them it may appear that the hot dog is shorter and that you're eating it in slow motion.

We'll return to this a bit later.

Today, as a segue from last week's simultaneity topic to this week's train examples, a railway version of the simultaneous events scenario.

This is a common Special Relativity thought experiment, one of three I'll discuss this week. This is a concrete version of the "simultaneity is relative" examples discussed all of last week.

(These Special Relativity examples often use trains. Perhaps because trains are somewhat one-dimensional — I mean long and skinny, not "shallow" — or perhaps because Einstein used them in his examples.)

As always, Em is speeding past Al (although she may not see it that way). This time she's in a train. And there's an electrical storm!

Diagram 1. Al's frame of reference. Em passes (in train) left to right.

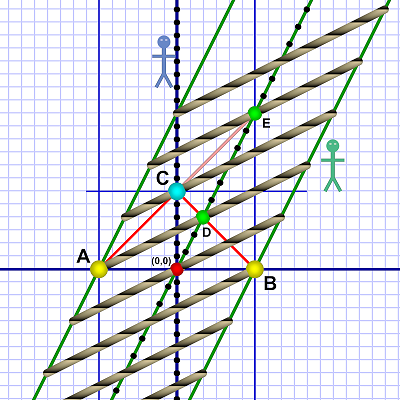
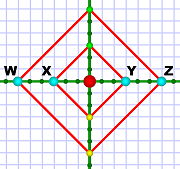
Al stands on the ground (safely sheltered) watching Em pass in a train (traveling at extremely high speed — 1/2 c). As the train passes, two lightning strikes occur (events A & B), one hits the front of the train, one hits the back.

Diagram 1 shows Al's frame of reference. His world line is the vertical center line (with timer-tick dots). Two other vertical lines, one on either side, mark the locations along the track where the lightning bolts hit.

Em is in the center of the passing train. Her world line — from Al's perspective — is the thick green line (also with timer-tick dots). She passes Al from (our) left to right. Green lines on either side of Em represent the back (left line) and front (right line) of the train — which is shown in a series of snapshots (the stripey things).

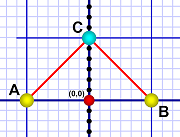Simultaneous

Al sees the lightning strikes (event C) as simultaneous. They are in his frame of reference and equal distance from him, so he sees both flashes at the same time. Even if the distances differed and Al saw the flashes separately, his analysis of the distances and times would show him the flashes occurred simultaneously in his frame of reference.

Em does see the lightning flashes separately (events D & E). As she stands at the midway of the train, she passes Al who is standing by the track. Both agree to call the moment and location (the event) they pass the origin, the (0x, 0t) point (as usual, the red dot).

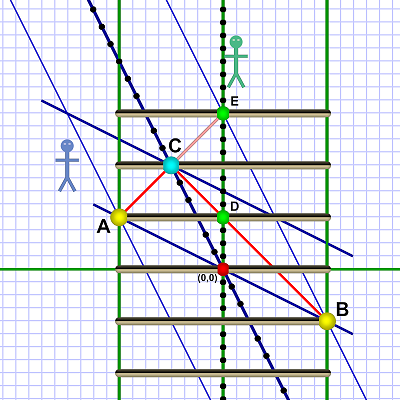
There's a lot to unpack here, but first let's see what Em's frame of reference looks like:

Diagram 2. Em's frame of reference (on train). Al passes right to left.

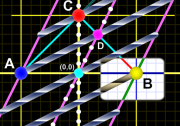
This time it's Al who seems going past (from right to left) while Em and the train stand still. Em is the vertical world line (center); vertical (green) lines to the left and right represent the back and front (respectively) of the train — which is shown again in a series of snapshots.

The events A-E are as described above, but their sequence, their timing, is different from Em's point of view. Any analysis she makes of the lightning flashes shows B  happening before A.

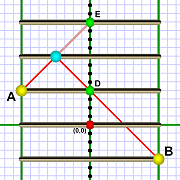Not Simultaneous

From Em's point of view, first, lightning hits the front of the train, then she passes Al (or Al passes her; same thing), lastly, lightning hits the back of the train.

Note that she sees the lightning flashes at D & E. (respectively her +4t and +12t). Her analysis of the flash she sees at D tells her lightning hit the front of the train at (her) t=-4. Likewise, she knows the flash she sees at E happened at the back of the train at her t=+4.

But Al's analysis shows A and B happened simultaneously at  the moment Em passed him.

The key lies in the lines of simultaneity. The train is drawn at a slant in diagram 1; that slant is the line of simultaneity for Em. As discussed last week, this line (surface, actually) shifts due to relative motion.

Symmetry

Think about Em standing midway along the train.

If she does the light-bounce simultaneity experiments we explored previously, she finds that all points along the train are in her frame of reference and share a clock.

She can measure distances on the train, and those tell her how long light takes to reach her eye from any given point. Thus she knows when anything she sees happens. She has, on the train, a space-time coordinate system.

Therefore, for Em, the train is a line of simultaneity (as shown in diagram 2). But when it is in motion relative to Al, he sees it as shifted from his lines of simultaneity.

B flash first

You can see in diagram 1 that, at the moment of lightning flash B, the front of the train has just reached that point.

The front of the train and flash B are in Al's line of simultaneity with the flash at A (and Al's origin point).

But Em is at the midway point of that particular snapshot of the train. She's below Al's horizontal (t=0) origin line — she hasn't passed him; for her it's still some "T-minus" time. In the next snapshot, when the mid-point of the train reaches the origin (red dot), the front of the train is past the B point (in time and in space).

A flash later

And the rear of the train still hasn't gotten to the flash A point! It does that in the following snapshot. Em is now above Al's origin line, so for her the flash at the back of the train occurs after she passes Al.

Compare these three snapshot moments to the three matching ones in diagram 2. The same events: the front of the train at B; the middle (with Em) at the origin t=0 line; the end at A.

In diagram 1 the moment (event C) Al sees both lightning flashes has a short line of simultaneity marking that moment (t=+6). Diagram 2 shows the same line (as well as, and above, Al's t=0 line). From Em's point of view, Al's lines of simultaneity are shifted, but in the opposite direction.

Essentially, a moving frame's coordinate system appears rotated to observers. For any given one of Al's lines of simultaneity, Em and the train are rotated out of that line.

The c Train

The train — in a sense — doesn't exist as a whole in that moment. The whole of the train passes through that moment, but not simultaneously.

The room you're sitting in, it's passing through time synchronously with you — the whole room exists with you in every given moment. But the passing train does not; it travels through your moments progressively, not as a whole.

Freaky, eh?

Next time I'll turn the situation around and look at how Al sees events on the passing train — events that Em, on the train, sees as simultaneous.